Currency and Price Stability

There are two related claims made by gold standard advocates. These claims are:


1) Under a gold standard, prices are stable.
2) Prices ought to remain stable.

I) We'll start by looking at the first claim. What does it mean to say that prices are stable?

One piece of evidence looked at is the purchasing power of an ounce of gold. The rule of thumb that is frequently referenced is that an ounce of gold can buy a good suit. Unfortunately I don't have a price series for good suits (if anyone does, it might make for an interesting study). One thing we can easily look at is the the price of gold in today's dollars (using CPI):

I'm reluctant to call that "stable". It looks to me to be a rather bumpy ride. We'll get back to that issue shortly.

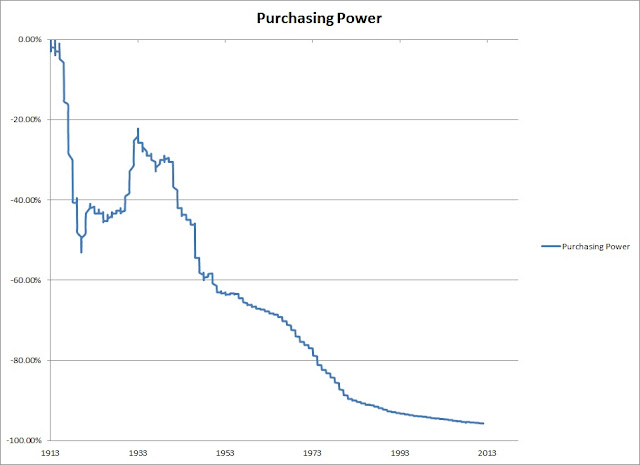
The second piece of evidence is that of the purchasing power of the dollar. Here's a glance at one such look (starting with the existence of the Fed in 1913):

Clearly the overall purchasing power of a dollar has declined significantly. But there's more to the story than just purchasing power. Let's look at year over year changes in CPI for different time periods:

I've selected the time periods to coincide with the existence of the Fed (1913), when FDR closed the gold window (1932) and the Nixon shock (1971).

We see early on, when the dollar was much more closely tied to gold, that the mean inflation rate was close to 0%. After we move off of the gold standard (partly in 1932, completely in 1971), we see that mean inflation is much higher. This is clearly not "stable" at least in the sense that most advocates of the gold standard refer.

But there's another interesting feature. Inflation was much more volatile during the periods when the dollar was more closely tied with gold. Standard deviation was quite high.

So what is "price stability"? I suspect what most people think of as price stability is that prices remain roughly the same over time. That suggests that the inflation rate should have both a mean and standard deviation both close to 0%.

The evidence above suggests that neither when the dollar was more closely tied to gold nor when it was decoupled from gold were prices "stable" in the above sense. The dollar (tied to gold) was "stable" in the sense that the mean CPI rate was close to 0% but quite volatile as far as how the rate varied. Contrast that with the dollar post-gold standard was "stable" in the sense that CPI rate varied much less but was still on average above 0%.

I will use the terms "mean stable" to refer to mean inflation rate being close to 0% and "volatility stable" to refer to the mean standard deviation being close to 0%. 

II) Let's take a brief look at the second question. Ought the price level be stable over time?

We've already encountered the issue of what is even meant by price stability. We first have to define our terms. What I'd like to offer is an argument why prices ought not be stable at least in the sense that mean price level should be 0%. This is less an argument in favor of that position but to merely challenge the implicit assumption that prices should remain stable over time.

To begin with let me start with a quote from a blog post defending the gold standard:

"To summarize in simple terms, a child with 4 cents in his pocket could buy the same amount of candy in 1913 as his descendant could with $1 in 2012." (from here).

I actually think the example is interesting. The supposition is that if I had 4 cents in 1913 and saved it until 2012, I should still be able to buy the "same amount" of candy. The author is not asserting that I should be able to buy the same  candy. After all, who would want to consume a piece of candy from 1913? (No doubt a collector might want it but a collector might also want 4 cents from 1913.)

The reality of the matter is that the piece of candy depreciated over time. Food spoils, steel rusts, technology becomes obsolete, the 2nd law of thermodynamics wears things down. As a result, things depreciate in value.

So if currency, which is sometimes alleged to represent (in some abstract sense) the value of what we produce, then why shouldn't our currency - much like that which we produce - depreciate over time?

My suggestion is that those who claim that prices ought to remain stable (mean stability) over time are basically claiming that something I produced 20 years ago (which I did not consume) ought to still be worth the same today. I see no reason to hold to this. If anything, I think there's reason to hold a "use it or lose it" policy. Either I need to consume what I produce, or put it to productive use; if not, then I shouldn't be surprised if it loses value.

Now there is something to be said for what might be called "volatility stability". If prices are so volatile that, as a business person, it becomes difficult to make decisions then I think we have a problem. The key, in my opinion, is not whether or not prices go up over time but whether or not they do so in a predictable way. If I can plan for price inflation then it's less of a problem.

In summary, I think that gold is price stable in the sense that it has mean stability. I see no evidence that a gold standard would offer volatility stability. So I guess in answer to the first question (is gold price stable?) the answer is a mixed "yes" and "no" depending upon what we're talking about. If we just mean "mean stability" then I think gold is price stable.

However, I see no reason to hold that prices ought to be mean price stable and would further argue that prices ought to increase over time.

On the other hand, I think there may be good reasons for having prices be "standard deviation stable" as that will make business calculation easier.

loading..