There's a metaphor that goes around talking about "picking up pennies in front of a steamroller". It's in reference to investment strategies in which the investor risks getting run over by a steamroller (taking the risk of getting wiped out) for the benefit of receiving a couple of pennies. This entire reference is to Downside Risk Investing.
Now in some sense, many investment strategies have the potential for huge losses with limited upside. The question we should be asking is this: how many pennies do I need to be able to pick up for a particular Downside Risk Investing to be a good strategy? I don't know if I have an answer to that question but I'd like to present a discussion. So without further ado, here are some examples of strategies that I think fit the bill (some of which may be good strategies to employ).
1. Bonds
Bonds are a loan from the bondholder to an organization. They make contractually obligated payments (e.g. they pay interest).
Upside:
The upside to bonds is the yield to maturity (YTM). If you buy and hold a bond until it matures, that's going to be the maximum return you're going to get.
Downside:
The primary risk associated with bonds is default risk. In the event that a company defaults, any number of events can occur such as liquidation of the company or restructuring. In such cases bond holders may only receive pennies on the dollar.
Comments:
This won't apply to bonds that have special features like convertibility. This gives the bond holder the opportunity to participate in the upside of the stock if it works out for them. As a result, potential rewards would be quite high.
Some of this will also apply to preferred shares who do not have any special features like convertibility. Preferred shares have a higher return but a lower recovery rate (e.g. more downside risk).
Here's a summary of Default Rates by Moody's credit rating from 1920-2007. (See here for full article).
Figure 1
Here's a list of Recovery Rates for the period between 1983-2008 from Moody's. (See here for full article).
Figure 2
Losses on bonds and preferred shares can be quite substantial in the event of a default.
2. Merger Arbitrage
Merger arbitrage occurs when one company (Company "A") decides to purchase another company (Company "B"). There are two ways this strategy is typically employed. If Company A is paying cash for Company B, the investor simply buys shares of Company B. If Company A is using its own stock to pay for Company B, the investor may buy shares of Company B and short sell shares of Company B.
Upside:
The upside is the difference between the current market price of company being acquired and the offer price of the deal. It often amounts to a small return but the time until the deal is often quite short.
Downside:
In some cases, the deal may fall through. In such a case, the stock of the company may decline in price. Such declines can be quite high relative to the potential reward of the deal.
Comments:
To give some perspective on Merger Aribtrage risk and returns, here is some summary information compiled from here and here.
Figure 3
Median Loss Potential represents the median loss in value that could occur if the price of the stock reverted to it's pre-acquisition announcement market price. Given that losses could be as high as 20-30% and potential returns are only about 2%, there's a good sized loss potential relative to overall returns.
Successful Deals represents the number of acquisitions which actually occurred as a percentage of acquisition offers made. Average success rate from 1990-2007 was about 92%.
3. Put Writing
A put option gives the buyer the right but not obligation to sell a financial asset at a specified price (referred to as the strike price). The seller of the option (often referred to as the "writer") has the obligation to buy the financial asset if the buyer chooses. The seller receives a premium for offering this right to the buyer. These put options have an expiration typically ranging from 1 week to a couple of years.
Upside:
The asset's price ends up above the strike price. In this case the put writer keeps the premium and the option expires. In addition, the put writer receives interest on any cash put as collateral in the event that the buyer wants to sell the financial asset.
Downside:
If the financial asset collapses in price, the put writer will take on the losses of that financial asset which can be quite large.
Comments:
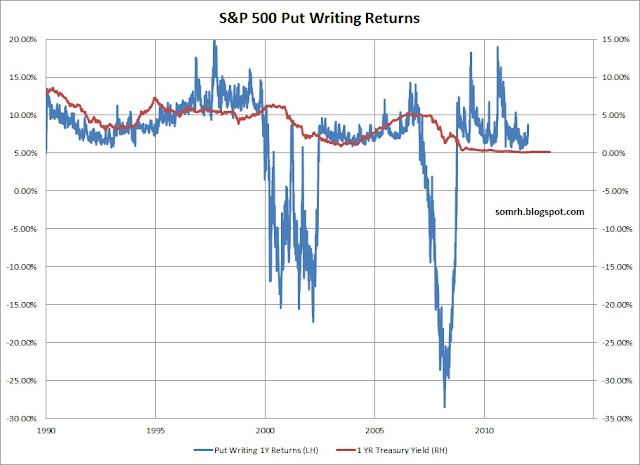
I've used the VIX index to construct a hypothetical year over year return for a put writing strategy on the S&P 500. While the strategy I used to construct the returns are not exactly replicable, it should give a rough idea of how returns might look.
Figure 4
Figure 5
| Summary Statistics | |
|---|---|
| Mean | 6.11% |
| Standard Deviation | 7.65% |
| Max | 19.95% |
| Min | -28.50% |
Approximately 81% of the returns fell within the 5-15% range. But some periods resulted in substantial losses. I think this does a decent job of exemplifying the risk-reward structure of Downside Risk Investing strategies.
4. Covered Call Writing
A covered call writing strategy involves buying stock and writing (selling) a call option on that stock. That call option gives the buyer the right, but not obligation, to buy your shares from you at the specified price (strike price).
According to Put-call parity, a covered call strategy has a similar risk-reward structure to a put writing strategy.
Upside:
The upside is restricted to the premium that is received for selling the call option, any dividends paid by the stock and any price appreciation that is between the buy price of the stock and the strike price.
Downside:
The downside is any decline in the price of the stock. In this case the options expire worthless but the call writer takes on the losses of the stock ownership.
Comments:
Risk and returns for this strategy has similarity to the put writing strategy discussed in #3.
5. Low Beta Stocks
Stocks, as a rule of thumb, don't exactly fit the Downside Risk category. Most stocks have solid upside potential. While a stock holder can lose up to 100% of the initial investment, stocks can become tenbaggers (a phrase Peter Lynch defined in his book One Up on Wall Street as a stock who increased in price by a factor of 10).
But what got me thinking about a lot of this is an article that comes from the good folks at GMO: Re-Thinking Risk: What the Beta Puzzle Tells Us about Investing.
The article discusses similarities between the risk/reward structure of put-writing, hedge fund returns and low beta stocks. They all exhibit what the authors call a "concave" profile versus other strategies such as high beta and call options which give a "convex" profile.
6. Credit Default Swaps
Credit default swaps are typically classified derivatives (I'm far more sympathetic to the insurance metaphor but that's neither here nor there). They pay out in the event that a bond defaults. CDS became popular in the media when AIG suffered losses on CDS they sold on mortgage related assets. The buyer has to pay a premium to the seller.
Upside:
The bond in question has no default event and the seller gets to keep the premium.
Downside:
The bond defaults and the seller has to settle an amount with the buyer as specified by the contract. The settlement can be either in cash (e.g. the difference between par value and present market value of the bond) or physical (e.g. the seller pays par value and receives the bond in question). The latter has some similarity to put writing strategies.
Comments:
I only have a basic understanding of credit default swaps so I'm not sure any analysis would have much value here. I suspect that the overall risk-reward structure of CDS will have similarities with bonds.
7. Insurance
I thought I'd include something different here partly because I think the comparison between insurance and CDS and partly because I think this takes on a similar risk/reward structure. While insurance covers a broad collection of policies (e.g. life insurance, home insurance, auto insurance, health insurance, business asset insurance, etc) the basic structure of most of these will fit the general Downside Risk Investing theme.
Upside:
The upside is that the insurance premiums will be paid and no claims on the policy will be made.
Downside:
Substantial claims are made and the insurance company has to pay on those claims. For example, a fire burns down a home and the insurance company pays an amount for rebuilding the home.
Comments:
The other reason to include insurance here is I suspect that actuarial techniques might offer some insight regarding how to analyze Downside Risk Investing strategies more generally.
Concluding Observations
While the above doesn't represent an exhaustive list of what I'm classifying as Downside Risk Investing, it should provide a decent sample of examples that illustrate the idea I have in mind.
So when is Downside Risk Investing a good investment strategy?
I don't know if I have an answer to that but I have general observations that might motivate an approach to analyzing whether the risk/reward structure of a particular strategy makes it a good opportunity or not.
1) Diversification
This probably seems like a no-brainer but there's a critical aspect to this: correlation.
Diversification will work better for some strategies than others. Take bonds for example. Bond default rates tend to cluster.
Figure 6
This makes sense of course. During recessions, demand for products decline, revenues fall off, margins tighten. This can put pressure on firms that are levered with debt.
Contrast that with the success/failure rates of merger arbitrage deals. As Figure 3 illustrates, the percentage of successful deals fell within the 80-98% and there weren't obvious clustering of successes/failures. That suggests that bond default are going to be more correlated than merger deal failures. As a result, merger arbitrage should be an easier strategy to diversify.
So I think there should be some preferential treatment to strategies that can be diversified with lower correlation between the different instances.
2) A Simple Model
One way we can model is this is by making a few assumptions. Here are the basic assumptions:
- On any given time period, there are two possible events: success or failure. We'll denote the probability of success as S.
- If the period results in a success, you'll receive a profit P. Otherwise you will receive a loss L.
$$E(X) = PS + L(1-S) $$
$$STD(X) = \sqrt{P^2S- P^2S^2-2PLS+2PLS^2+L^2S-L^2S^2 }$$
Then one can see whether the overall return is adequate for the risk. Here's a couple of examples:
Example 1: Bond earning 6% (P = 6%) with a annual default rate of 1% (S = 99%) and recovery rate of 40% (L = -60%). This gives us:
$$E(X) = 5.34 \%$$
$$STD(X) = 6.57 \%$$
Example 2: A merger arbitrage opportunity with an offer spread of 2% (P = 2%), success rate of 92% (S = 92%) and a potential loss of 20% (L = -20%).
$$E(X) = 0.24 \%$$
$$STD(X) = 5.97 \%$$
Of course the merger arbitrage opportunity might go through in, say, 4 months time. So annualized, the expected return might be around 0.72%.
Due to spreads being currently low, I wouldn't expect great returns from merger arbitrage now based upon the above simple model. But they may be adequate given that they are low duration and due to the fact that low maturity interest rates are quite low.
Obviously the above model will not work as well for something like a put-writing strategy.
In any event, I'd like to explore this idea further and to attempt to see where the risk/reward profile of these strategies offers an adequate return.
Edit:
I can't believe I didn't notice that CBOE publishes their index data. So here's an overall look at my put option strategy (Figure 4) along with CBOE's Put-write index and Buy-write index.
Figure 7
CBOE's Put-Write and Buy-Write strategies offer a similar risk-reward structure (due to put-call parity mentioned above).
No comments:
Post a Comment
Some common OpenID URLs (no change to URL required):
Google: https://www.google.com/accounts/o8/id
Yahoo: http://me.yahoo.com/